This is one of the following sixteen articles on Single-Factor ANOVA in Excel

Overview Post-Hoc

Testing For Single-Factor

ANOVA

The F-test in ANOVA is classified as an omnibus test. An omnibus test is one that-Tests the overall significance of a model to determine whether a difference exists but not exactly where the difference is. ANOVA test the Null Hypothesis that all of the group means are the same. When this Null Hypothesis is rejected, further testing must be performed to determine which pairs of means are significantly different. That type of testing is called Post-Hoc testing.

Post-Hoc testing is a pairwise comparison. Groups means are compared two at a time to determine whether the difference between the pair of means is significant.

The Many Types of Post-Hoc Tests

Available

There are many types of Post-Hoc tests available in most major statistical software packages but two have become the preferred tests. These two are the Tukey-Kramer test and the Games-Howell test. The Tukey-Kramer test should be used when group variances are similar. The Games-Howell test should be used with group variances are dissimilar. Both tests can be used when group sizes are unequal.

The Tukey-Kramer test is a slight variation of the well-known Tukey HSD test. The Tukey-Kramer can be used when group sizes are unequal, which the Tukey HSD test is not designed for.

Post-Hoc Tests Used When Group Variances Are Equal

SPSS lists the following Post-Hoc tests or corrections available when groups variances are equal:

LSD

Bonferroni

Sidak

Scheffe

REGWF

REGWQ

S-N-K

Tukey (Tukey’s HSD or Tukey-Kramer)

Tukey’s b

Duncan

Hochberg’s GT2

Gabriel

Waller-Duncan

Dunnett

Of all of the Post-Hoc tests available when groups variances are found to be similar, Tukey’s HSD test is used much more often than the others. A slight variation of Tukey’s HSD called the Tukey-Kramer test is normally used when group variances are the same but group sample sizes are different. Tukey’s HSD can only be used when group sizes are exactly the same.

The Tukey test (Tukey’s HSD test or the Tukey-Kramer test) is generally a good choice when group variances are similar. Hochberg’s GT2 produces the best result when group sizes are very different. REGWQ is slightly more accurate than the Tukey test but should only be used when group sizes are the same.

Post-Hoc Tests Used When Group Variances Are Not Equal

SPSS lists the following Post-Hoc tests available when groups variances are not equal:

Tamhane’s T2

Dunnett’s T3

Games-Howell

Dunnett’s C

The Games-Howell test is the most widely used of the above and is generally a good choice when group variances are not similar. The Games-Howell test can be used when group sizes are not the same.

Tukey’s HSD (Honestly Significant Difference) Test

Used When Group Sizes and Group Variances Are Equal

Tukey’s HSD test compares the difference between each pair of group means to determine which differences are large enough to be considered significant.

Tukey’s HSD test is very similar to a t-test except that it makes a correction for the experiment-wide error rate that a t-test doesn’t. The experiment-wide error rate is the increased probability of type 1 errors (false positives) when multiple comparisons are made.

Tukey’s HSD test can be summarized as follows:

The means of all groups are arranged into as many unique pair combinations as possible. The pair combination with the largest difference between the two means is tested first. A test statistic for this pair of means is calculated as follows:

(Click Image To See a Larger Version)

where

(Click Image To See a Larger Version)

n = number of samples in any group (all groups must be of equal size for Tukey’s HSD Post-Hoc test)

This test statistic is compared to q_Critical. The critical q values are found on the Studentized Range q table. A unique critical q value is calculated for each unique combination of level of significance (usually set at 0.05), the degrees of freedom, and the total number of groups in the ANOVA analysis.

Tukey’s test calculates degrees of freedom as follows:

df = Degrees of freedom = (total number of samples in all groups combined) – (total number of groups)

The difference between the two means is designated as significant if its test statistic q is larger than the critical q value from the table.

If the difference between the means with the largest difference is found to be significant, the next inside pair of means is tested. This step is repeated until an innermost pair is found to have a difference that is not significant. Once an inner pair of means is found to have a difference that is not large enough to be significant, no further testing needs to be done because all untested pairs will be inside this one and have even smaller differences between the means.

Tukey-Kramer Test

Used When Group Variances Are Equal But Group Sizes Are Unequal

A slightly variation of Tukey’s HSD test should be used when group sizes are not the same. This variation of Tukey’s HSD test is called the Tukey-Kramer test.

This Tukey-Kramer test will normally be performed instead Tukey’s HSD test by most statistical packages. The Tukey-Kramer test produces the same answer as Tukey’s HSD when group sizes are the same and can be used when group sizes are different (unlike Tukey’s HSD).

Recall that the Tukey’s HSD test statistic for a pair of means is calculated as follows:

(Click Image To See a Larger Version)

where

(Click Image To See a Larger Version)

The Tukey-Kramer test makes the following adjustment to standard error to account for unequal group sizes. The pooled variance MS_{Within_Groups} is multiplied by the average of ( 1/n_i+ 1/n_j ) instead of 1/n.

(Click Image To See a Larger Version)

As with Tukey’s HSD test, the Tukey-Kramer test calculates Test Statistic q for each pair of means. This Test Statistic is compared to q_Critical. The critical q values are found on the Studentized Range q table. A unique critical q value is calculated for each unique combination of level of significance (usually set at 0.05), the degrees of freedom, and the total number of groups in the ANOVA analysis. An Excel lookup function can be conveniently used to obtain the critical q value. The easiest Excel lookup function in this case is Index(array, row, column).

The Tukey-Kramer test calculates degrees of freedom in the same way as Tukey’s HSD test as follows:

df = Degrees of freedom = (total number of samples) – (total number of groups)

The difference between the two means is designated as significant if its test statistic q is larger than the critical q value from the table.

The Tukey-Kramer test will be performed on the sample data shortly.

Games-Howell Test

Used When Groups Variances Are Not Equal. Groups Sizes Can Be Unequal.

The Games-Howell test is the Post-Hoc test used when group variances cannot be confirmed to be homogeneous (similar). The Games-Howell test can be used whether or not sample sizes are the same.

When group variances are shown to be dissimilar, the Single-factor ANOVA test should be replaced by either Welch’s ANOVA or the Brown-Forsythe F-Test. Both of these tests will be performed on the sample data at the end of this chapter.

The two main tests used to evaluate samples for homogeneity (sameness) of variance are Levene’s test and the Brown-Forsythe test.

Levene’s test is an ANOVA test the compares distances between sample values and group means.

The Brown-Forsythe test is an ANOVA test that compares distances between sample values and group medians. The Brown-Forsythe test is more robust because it is less affected by outliers since it is based on the median and not the mean as Levene’s test is.

The F-test is not a good test to compare variances because it is extremely sensitive to non-normality of sample data.

The Games-Howell Post-Hoc test is performed in the same manner as Tukey’ HSD and the Tukey-Kramer test. The only differences are the formulas used to calculate standard error and the degrees of freedom.

Recall that the Tukey’s HSD test statistic for a pair of means is calculated as follows:

(Click Image To See a Larger Version)

where

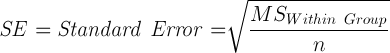
(Click Image To See a Larger Version)

Notice that both Tukey’s HSD and the Tukey-Kramer test use a pooled variance MS_{Within_Groups} because groups variances are similar. The Games-Howell test assumes dissimilar groups variances and calculates the standard error using individual variances of each group as follows:

(Click Image To See a Larger Version)

The Games-Howells test calculates degrees of freedom in a different way as well. The formula for df is as follows:

(Click Image To See a Larger Version)

In Excel terms, the formula is expressed as the following:

df = ( ( (Var₁/n₁) + (Var₂/n₂) )^2 ) / ( ((Var₁/n₁)^2 / (n₁ - 1) ) + ( (Var₂/n₂)^2 / (n₂-1) } )

This is the same formula used to calculate degrees of freedom for a two-independent sample, unpooled t-test. This t-test is known as Welch’s t-test. As mentioned, when group variances are unequal, Single-Factor ANOVA is replaced by Welch’s ANOVA or the Brown-Forsythe F-Test.

As with Tukey’s HSD test and the Tukey-Kramer test, the Games-Howell test calculates Test Statistic q for each pair of means. This Test Statistic is compared to q_Critical. The critical q values are found on the Studentized Range q table. A unique critical q value is calculated for each unique combination of level of significance (usually set at 0.05), the degrees of freedom, and the total number of groups in the ANOVA analysis. An Excel lookup function can be conveniently used to obtain the critical q value. The easiest Excel lookup function in this case is Index(array, row, column).

The difference between the two means is designated as significant if its test statistic q is larger than the critical q value from the table.

The Games-Howell test will be performed on the sample data shortly.

Excel Master Series Blog Directory

Statistical Topics and Articles In Each Topic

Technorati Tags: post hoc,anova statistics,excel,excel 2010,excel 2013,tukey,tukey's,tukeys,games-howell

Become an Excel Statistical Master

Excel Master Series - MBA-level statistics - Over 1,100+ Pages of Easy-To-Follow Instructions in Excel

It's a Full
Easy-To-Follow
MBA Course in Business Statistics

ALL IN EXCEL

&

MUCH Clearer

Than Your Text

Book

Download the
1,100+ Page Excel Statistical Master now

More Easy-To-

Follow eManuals

That You Will

Master Quickly

Step-By-Step Optimization With Excel Solver

What's In It?

For anyone who wants to be operating at a high level with the Excel Solver quickly, this is the book for you. Step-By-Step Optimization With Excel Solver is a 200+ page .pdf e-manual of simple yet thorough explanations on how to use the Excel Solver to solve today’s most widely known optimization problems. Loaded with screen shots that are coupled with easy-to-follow instructions, this book will simplify many difficult optimization problems and make you a master of the Excel Solver almost immediately.

Here are just some of the Solver optimization problems that are solved completely with simple-to-understand instructions and screen shots in this e-manual:

• The famous “Traveling Salesman” problem using Solver’s Alldifferent constraint and the Solver’s Evolutionary method to find the shortest path to reach all customers. This also provides an advanced use of the Excel INDEX function.

• The well-known “Knapsack Problem” which shows how optimize the use of limited space while satisfying numerous other criteria.

• How to perform nonlinear regression and curve-fitting on the Solver using the Solver’s GRG Nonlinear solving method.

• How to solve the “Cutting Stock Problem” faced by many manufacturing companies who are trying to determine the optimal way to cut sheets of material to minimize waste while satisfying customer orders.

• Portfolio optimization to maximize return or minimize risk.

• Venture capital investment selection using the Solver’s Binary constraint to maximize Net Present Value of selected cash flows at year 0. Clever use of the If-Then-Else statements makes this a simple problem.

• How use Solver to minimize the total cost of purchasing and shipping goods from multiple suppliers to multiple locations.

• How to optimize the selection of different production machine to minimize cost while fulfilling an order.

• How to optimally allocate a marketing budget to generate the greatest reach and frequency or number of inbound leads at the lowest cost.

Step-By-Step Optimization With Excel Solver has complete instructions and numerous tips on every aspect of operating the Excel Solver. You’ll fully understand the reports and know exactly how to tweek all of the Solver’s settings for total custom use. This e-manual also provides lots of inside advice and guidance on setting up the model in Excel so that it will be as simple and intuitive as possible to work with.

All of the optimization problems in this book are solved step-by-step using a 6-step process that works every time. In addition to detailed screen shots and easy-to-follow explanations on how to solve every optimization problem in the book, a link is provided to download an Excel workbook that has all problems completed exactly as they are in this e-manual.

Step-By-Step Optimization With Excel Solver is exactly the e-manual you need if you want to be optimizing at an advanced level with the Excel Solver quickly.

*******************

Become an Excel Statistical Master

It's a Full
Easy-To-Follow
MBA Course in Business Statistics

ALL IN EXCEL

&

MUCH Clearer

Than Your Text

Book

Download the
1,100+ Page Excel Statistical Master now

More Easy-To-

Follow eManuals

That You Will

Master Quickly

*******************

Become an Excel Statistical Master

It's a Full
Easy-To-Follow
MBA Course in Business Statistics

ALL IN EXCEL

&

MUCH Clearer

Than Your Text

Book

Download the
1,100+ Page Excel Statistical Master now

More Easy-To-

Follow eManuals

That You Will

Master Quickly

*******************

Become an Excel Statistical Master

It's a Full
Easy-To-Follow
MBA Course in Business Statistics

ALL IN EXCEL

&

MUCH Clearer

Than Your Text

Book

Download the
1,100+ Page Excel Statistical Master now

Immediate, Absolute, No-Questions-Asked, Money-Back Guarantee If Not TOTALLY, 100% Satisfied. In Other Words, If Any Excel Master Series eManual That You've Purchased Here Does Not Provide Instructions That Are CRYSTAL CLEAR and EASY TO UNDERSTAND, You Get All Of Your Money Back Immediately and Keep the eManual. Guaranteed!

Meet The Author