This is one of the following sixteen articles on Single-Factor ANOVA in Excel
Overview of Single-Factor ANOVA
Single-Factor ANOVA in 5 Steps in Excel 2010 and Excel 2013
Shapiro-Wilk Normality Test in Excel For Each Single-Factor ANOVA Sample Group
Kruskal-Wallis Test Alternative For Single Factor ANOVA in 7 Steps in Excel 2010 and Excel 2013
Levene’s and Brown-Forsythe Tests in Excel For Single-Factor ANOVA Sample Group Variance Comparison
Single-Factor ANOVA - All Excel Calculations
Overview of Post-Hoc Testing For Single-Factor ANOVA
Tukey-Kramer Post-Hoc Test in Excel For Single-Factor ANOVA
Games-Howell Post-Hoc Test in Excel For Single-Factor ANOVA
Overview of Effect Size For Single-Factor ANOVA
ANOVA Effect Size Calculation Eta Squared in Excel 2010 and Excel 2013
ANOVA Effect Size Calculation Psi – RMSSE – in Excel 2010 and Excel 2013
ANOVA Effect Size Calculation Omega Squared in Excel 2010 and Excel 2013
Power of Single-Factor ANOVA Test Using Free Utility G*Power
Kruskal-Wallis Test in 7
Steps as Nonparametric
Alternative For Single-
Factor ANOVA in Excel
Single-Factor ANOVA requires that the samples are taken from normally-distributed populations. If the populations are normally-distributed, the samples will be normally-distributed if the sample size is large enough, i.e., each sample contains at 15 to 20 data points.
If normality tests indicate that the samples are likely not normally-distributed, the nonparametric Kruskal-Wallis test should be substituted for Single-Factor ANOVA. The Kruskal-Wallis test is based upon the rankings of all data points and does not require that the data be normally-distributed.
The Kruskal-Wallis test does have a requirement that the data samples have similar distribution shapes. The Excel histogram is a convenient tool to quickly view the distribution shape of each sample group.
Excel histograms will be created for each sample group of the original data set. The original data set was already successfully tested for normality using the Shapiro-Wilk normality test. Excel histograms would therefore be expected to resemble the bell-shaped normal distribution curve. Histograms of each of the three data groups are shown in the following diagram:

(Click On Image To See a Larger Version)
This histogram was created in Excel by inputting the following information into the histogram dialogue box:
 (Click On Image To See a Larger Version)
(Click On Image To See a Larger Version)

(Click On Image To See a Larger Version)
This histogram was created in Excel by inputting the following information into the histogram dialogue box:

 (Click On Image To See a Larger Version)
(Click On Image To See a Larger Version)
This histogram was created in Excel by inputting the following information into the histogram dialogue box:
 (Click On Image To See a Larger Version)
(Click On Image To See a Larger Version)
Excel histograms of each of the data groups reveal similar distribution shapes thus validating this required assumption of the Kruskal-Wallis test.
The Kruskall-Wallis test is based upon the overall rankings of each data point. The sum of the rankings for each sample groups, Ri, is used to calculate the value of test statistic H as follows:

(Click On Image To See a Larger Version)
k = the number of sample groups
Test statistic H is very nearly distributed as the Chi-Square distribution with k – 1 degrees of freedom as long as the number of samples in each group is at least 5.
A p Value can therefore be derived from H as follows:
p value = CHISQ.DIST.RT(H, k-1)
If the p Value is smaller than the designated Level of Significance (Alpha is usually set at 0.05) then at least one of the groups has a disproportionately large share of higher numbers. A larger-than-expected share of higher numbers will produce an unexpectedly large rank sum, Ri, for the sample group. This will result in the small p Value which indicates that the difference between the rankings within sample groups is significant.
Kruskal-Wallis Test In Excel
The Kruskal-Wallis test is performed on the original sample data as follows:

(Click On Image To See a Larger Version)
Step 1 – Arrange All Data In One Column

(Click On Image To See a Larger Version)

(Click On Image To See a Larger Version)

(Click On Image To See a Larger Version)
Step 2 - Sort and Then Rank the Data Column.
The data sort must keep the group number attached to each data value.
 (Click On Image To See a Larger Version)
(Click On Image To See a Larger Version)
 (Click On Image To See a Larger Version)
(Click On Image To See a Larger Version)
 (Click On Image To See a Larger Version)
(Click On Image To See a Larger Version)
Step 3 – Take Care of Tied Data Values
Data whose values have ties are all assigned the same rank. This rank is the average rank that all of the same data would. This is calculation is performed as follows:
 (Click On Image To See a Larger Version)
(Click On Image To See a Larger Version)

Step 4 – Return Data To Original Groups
The data are then resorted back into their original group. The sort must retain the ranking for each data point.

(Click On Image To See a Larger Version)

(Click On Image To See a Larger Version)
Step 5) Calculate Rank Sum For Each Group
Calculate the Rank Sum for each data group by adding the rankings of all data points in the group.

(Click On Image To See a Larger Version)
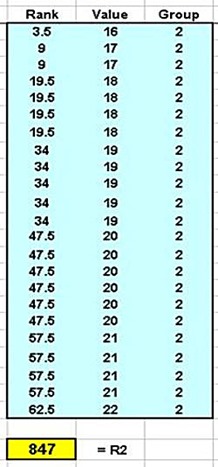
(Click On Image To See a Larger Version)

(Click On Image To See a Larger Version)
Using RANK.AVG() To Rank the Data
The data can be ranked and R calculated for each group much easier and quicker by using the RANK.AVG() formula as follows. Cell JF contains the following formula:
=IF(JD74=””,””,RANK.AVG($JD74,$JD$73:$JD$228,1))
 (Click On Image To See a Larger Version)
(Click On Image To See a Larger Version)
 (Click On Image To See a Larger Version)
(Click On Image To See a Larger Version)
 (Click On Image To See a Larger Version)
(Click On Image To See a Larger Version)
Step 6 – Calculate Test Statistic
Calculate test statistic based upon the following formula:

(Click On Image To See a Larger Version)
Ri = Rank Sum for group i
ni = number of data points in group i
n = the total number of data points in all groups
 (Click On Image To See a Larger Version)
(Click On Image To See a Larger Version)
Step 7 – Calculate the p Value
Calculate the p Value based upon h and k, the number of group as follows:

(Click On Image To See a Larger Version)
The p Value formula shown here is used in Excel versions prior to 2010. The equivalent formula in Excel 2010 and later is the following:
p Value = CHISQ.DIST.RT(H, df)
This Kruskal-Wallis test does not show (just barely) a significant difference between the rankings of the sample groups. The Kruskal-Wallis test is less sensitive than Single-Factor ANOVA. This is usually the case with any nonparametric test that is used to replace a parametric test.
In this case, the Kruskal-Wallis test shows a higher chance of a type 2 error than Single-Factor ANOVA. A type 2 error is a false negative. In other words, the Kruskal-Wallis test (p value = 0.0542) is less able to detect a significant difference than Single-Factor ANOVA (p value = 0.0369), Welch’s ANOVA (p Value = 0.0463), or the Brown-Forsythe F-test (p value = 0.0378).
Welch’s ANOVA Test and the Brown-Forsythe F Tests are performed on the sample data in the 11th and 12th blog articles beyond this
Excel Master Series Blog Directory
Statistical Topics and Articles In Each Topic
- Histograms in Excel
- Bar Chart in Excel
- Combinations & Permutations in Excel
- Normal Distribution in Excel
- Overview of the Normal Distribution
- Normal Distribution’s PDF (Probability Density Function) in Excel 2010 and Excel 2013
- Normal Distribution’s CDF (Cumulative Distribution Function) in Excel 2010 and Excel 2013
- Solving Normal Distribution Problems in Excel 2010 and Excel 2013
- Overview of the Standard Normal Distribution in Excel 2010 and Excel 2013
- An Important Difference Between the t and Normal Distribution Graphs
- The Empirical Rule and Chebyshev’s Theorem in Excel – Calculating How Much Data Is a Certain Distance From the Mean
- Demonstrating the Central Limit Theorem In Excel 2010 and Excel 2013 In An Easy-To-Understand Way
- t-Distribution in Excel
- Binomial Distribution in Excel
- z-Tests in Excel
- Overview of Hypothesis Tests Using the Normal Distribution in Excel 2010 and Excel 2013
- One-Sample z-Test in 4 Steps in Excel 2010 and Excel 2013
- 2-Sample Unpooled z-Test in 4 Steps in Excel 2010 and Excel 2013
- Overview of the Paired (Two-Dependent-Sample) z-Test in 4 Steps in Excel 2010 and Excel 2013
- t-Tests in Excel
- Overview of t-Tests: Hypothesis Tests that Use the t-Distribution
- 1-Sample t-Tests in Excel
- 1-Sample t-Test in 4 Steps in Excel 2010 and Excel 2013
- Excel Normality Testing For the 1-Sample t-Test in Excel 2010 and Excel 2013
- 1-Sample t-Test – Effect Size in Excel 2010 and Excel 2013
- 1-Sample t-Test Power With G*Power Utility
- Wilcoxon Signed-Rank Test in 8 Steps As a 1-Sample t-Test Alternative in Excel 2010 and Excel 2013
- Sign Test As a 1-Sample t-Test Alternative in Excel 2010 and Excel 2013
- 2-Independent-Sample Pooled t-Tests in Excel
- 2-Independent-Sample Pooled t-Test in 4 Steps in Excel 2010 and Excel 2013
- Excel Variance Tests: Levene’s, Brown-Forsythe, and F Test For 2-Sample Pooled t-Test in Excel 2010 and Excel 2013
- Excel Normality Tests Kolmogorov-Smirnov, Anderson-Darling, and Shapiro Wilk Tests For Two-Sample Pooled t-Test
- Two-Independent-Sample Pooled t-Test - All Excel Calculations
- 2- Sample Pooled t-Test – Effect Size in Excel 2010 and Excel 2013
- 2-Sample Pooled t-Test Power With G*Power Utility
- Mann-Whitney U Test in 12 Steps in Excel as 2-Sample Pooled t-Test Nonparametric Alternative in Excel 2010 and Excel 2013
- 2- Sample Pooled t-Test = Single-Factor ANOVA With 2 Sample Groups
- 2-Independent-Sample Unpooled t-Tests in Excel
- 2-Independent-Sample Unpooled t-Test in 4 Steps in Excel 2010 and Excel 2013
- Variance Tests: Levene’s Test, Brown-Forsythe Test, and F-Test in Excel For 2-Sample Unpooled t-Test
- Excel Normality Tests Kolmogorov-Smirnov, Anderson-Darling, and Shapiro-Wilk For 2-Sample Unpooled t-Test
- 2-Sample Unpooled t-Test Excel Calculations, Formulas, and Tools
- Effect Size for a 2-Independent-Sample Unpooled t-Test in Excel 2010 and Excel 2013
- Test Power of a 2-Independent Sample Unpooled t-Test With G-Power Utility
- Paired (2-Sample Dependent) t-Tests in Excel
- Paired t-Test in 4 Steps in Excel 2010 and Excel 2013
- Excel Normality Testing of Paired t-Test Data
- Paired t-Test Excel Calculations, Formulas, and Tools
- Paired t-Test – Effect Size in Excel 2010, and Excel 2013
- Paired t-Test – Test Power With G-Power Utility
- Wilcoxon Signed-Rank Test in 8 Steps As a Paired t-Test Alternative
- Sign Test in Excel As A Paired t-Test Alternative
- Hypothesis Tests of Proportion in Excel
- Hypothesis Tests of Proportion Overview (Hypothesis Testing On Binomial Data)
- 1-Sample Hypothesis Test of Proportion in 4 Steps in Excel 2010 and Excel 2013
- 2-Sample Pooled Hypothesis Test of Proportion in 4 Steps in Excel 2010 and Excel 2013
- How To Build a Much More Useful Split-Tester in Excel Than Google's Website Optimizer
- Chi-Square Independence Tests in Excel
- Chi-Square Goodness-Of-Fit Tests in Excel
- F Tests in Excel
- Correlation in Excel
- Pearson Correlation in Excel
- Spearman Correlation in Excel
- Confidence Intervals in Excel
- z-Based Confidence Intervals of a Population Mean in 2 Steps in Excel 2010 and Excel 2013
- t-Based Confidence Intervals of a Population Mean in 2 Steps in Excel 2010 and Excel 2013
- Minimum Sample Size to Limit the Size of a Confidence interval of a Population Mean
- Confidence Interval of Population Proportion in 2 Steps in Excel 2010 and Excel 2013
- Min Sample Size of Confidence Interval of Proportion in Excel 2010 and Excel 2013
- Simple Linear Regression in Excel
- Overview of Simple Linear Regression in Excel 2010 and Excel 2013
- Complete Simple Linear Regression Example in 7 Steps in Excel 2010 and Excel 2013
- Residual Evaluation For Simple Regression in 8 Steps in Excel 2010 and Excel 2013
- Residual Normality Tests in Excel – Kolmogorov-Smirnov Test, Anderson-Darling Test, and Shapiro-Wilk Test For Simple Linear Regression
- Evaluation of Simple Regression Output For Excel 2010 and Excel 2013
- All Calculations Performed By the Simple Regression Data Analysis Tool in Excel 2010 and Excel 2013
- Prediction Interval of Simple Regression in Excel 2010 and Excel 2013
- Multiple Linear Regression in Excel
- Basics of Multiple Regression in Excel 2010 and Excel 2013
- Complete Multiple Linear Regression Example in 6 Steps in Excel 2010 and Excel 2013
- Multiple Linear Regression’s Required Residual Assumptions
- Normality Testing of Residuals in Excel 2010 and Excel 2013
- Evaluating the Excel Output of Multiple Regression
- Estimating the Prediction Interval of Multiple Regression in Excel
- Regression - How To Do Conjoint Analysis Using Dummy Variable Regression in Excel
- Logistic Regression in Excel
- Logistic Regression Overview
- Logistic Regression in 6 Steps in Excel 2010 and Excel 2013
- R Square For Logistic Regression Overview
- Excel R Square Tests: Nagelkerke, Cox and Snell, and Log-Linear Ratio in Excel 2010 and Excel 2013
- Likelihood Ratio Is Better Than Wald Statistic To Determine if the Variable Coefficients Are Significant For Excel 2010 and Excel 2013
- Excel Classification Table: Logistic Regression’s Percentage Correct of Predicted Results in Excel 2010 and Excel 2013
- Hosmer- Lemeshow Test in Excel – Logistic Regression Goodness-of-Fit Test in Excel 2010 and Excel 2013
- Single-Factor ANOVA in Excel
- Overview of Single-Factor ANOVA
- Single-Factor ANOVA in 5 Steps in Excel 2010 and Excel 2013
- Shapiro-Wilk Normality Test in Excel For Each Single-Factor ANOVA Sample Group
- Kruskal-Wallis Test Alternative For Single Factor ANOVA in 7 Steps in Excel 2010 and Excel 2013
- Levene’s and Brown-Forsythe Tests in Excel For Single-Factor ANOVA Sample Group Variance Comparison
- Single-Factor ANOVA - All Excel Calculations
- Overview of Post-Hoc Testing For Single-Factor ANOVA
- Tukey-Kramer Post-Hoc Test in Excel For Single-Factor ANOVA
- Games-Howell Post-Hoc Test in Excel For Single-Factor ANOVA
- Overview of Effect Size For Single-Factor ANOVA
- ANOVA Effect Size Calculation Eta Squared in Excel 2010 and Excel 2013
- ANOVA Effect Size Calculation Psi – RMSSE – in Excel 2010 and Excel 2013
- ANOVA Effect Size Calculation Omega Squared in Excel 2010 and Excel 2013
- Power of Single-Factor ANOVA Test Using Free Utility G*Power
- Welch’s ANOVA Test in 8 Steps in Excel Substitute For Single-Factor ANOVA When Sample Variances Are Not Similar
- Brown-Forsythe F-Test in 4 Steps in Excel Substitute For Single-Factor ANOVA When Sample Variances Are Not Similar
- Two-Factor ANOVA With Replication in Excel
- Two-Factor ANOVA With Replication in 5 Steps in Excel 2010 and Excel 2013
- Variance Tests: Levene’s and Brown-Forsythe For 2-Factor ANOVA in Excel 2010 and Excel 2013
- Shapiro-Wilk Normality Test in Excel For 2-Factor ANOVA With Replication
- 2-Factor ANOVA With Replication Effect Size in Excel 2010 and Excel 2013
- Excel Post Hoc Tukey’s HSD Test For 2-Factor ANOVA With Replication
- 2-Factor ANOVA With Replication – Test Power With G-Power Utility
- Scheirer-Ray-Hare Test Alternative For 2-Factor ANOVA With Replication
- Two-Factor ANOVA Without Replication in Excel
- Randomized Block Design ANOVA in Excel
- Repeated-Measures ANOVA in Excel
- Single-Factor Repeated-Measures ANOVA in 4 Steps in Excel 2010 and Excel 2013
- Sphericity Testing in 9 Steps For Repeated Measures ANOVA in Excel 2010 and Excel 2013
- Effect Size For Repeated-Measures ANOVA in Excel 2010 and Excel 2013
- Friedman Test in 3 Steps For Repeated-Measures ANOVA in Excel 2010 and Excel 2013
- ANCOVA in Excel
- Normality Testing in Excel
- Creating a Box Plot in 8 Steps in Excel
- Creating a Normal Probability Plot With Adjustable Confidence Interval Bands in 9 Steps in Excel With Formulas and a Bar Chart
- Chi-Square Goodness-of-Fit Test For Normality in 9 Steps in Excel
- Kolmogorov-Smirnov, Anderson-Darling, and Shapiro-Wilk Normality Tests in Excel
- Nonparametric Testing in Excel
- Mann-Whitney U Test in 12 Steps in Excel
- Wilcoxon Signed-Rank Test in 8 Steps in Excel
- Sign Test in Excel
- Friedman Test in 3 Steps in Excel
- Scheirer-Ray-Hope Test in Excel
- Welch's ANOVA Test in 8 Steps Test in Excel
- Brown-Forsythe F Test in 4 Steps Test in Excel
- Levene's Test and Brown-Forsythe Variance Tests in Excel
- Chi-Square Independence Test in 7 Steps in Excel
- Chi-Square Goodness-of-Fit Tests in Excel
- Chi-Square Population Variance Test in Excel
- Post Hoc Testing in Excel
- Creating Interactive Graphs of Statistical Distributions in Excel
- Interactive Statistical Distribution Graph in Excel 2010 and Excel 2013
- Interactive Graph of the Normal Distribution in Excel 2010 and Excel 2013
- Interactive Graph of the Chi-Square Distribution in Excel 2010 and Excel 2013
- Interactive Graph of the t-Distribution in Excel 2010 and Excel 2013
- Interactive Graph of the t-Distribution’s PDF in Excel 2010 and Excel 2013
- Interactive Graph of the t-Distribution’s CDF in Excel 2010 and Excel 2013
- Interactive Graph of the Binomial Distribution in Excel 2010 and Excel 2013
- Interactive Graph of the Exponential Distribution in Excel 2010 and Excel 2013
- Interactive Graph of the Beta Distribution in Excel 2010 and Excel 2013
- Interactive Graph of the Gamma Distribution in Excel 2010 and Excel 2013
- Interactive Graph of the Poisson Distribution in Excel 2010 and Excel 2013
- Solving Problems With Other Distributions in Excel
- Solving Uniform Distribution Problems in Excel 2010 and Excel 2013
- Solving Multinomial Distribution Problems in Excel 2010 and Excel 2013
- Solving Exponential Distribution Problems in Excel 2010 and Excel 2013
- Solving Beta Distribution Problems in Excel 2010 and Excel 2013
- Solving Gamma Distribution Problems in Excel 2010 and Excel 2013
- Solving Poisson Distribution Problems in Excel 2010 and Excel 2013
- Optimization With Excel Solver
- Maximizing Lead Generation With Excel Solver
- Minimizing Cutting Stock Waste With Excel Solver
- Optimal Investment Selection With Excel Solver
- Minimizing the Total Cost of Shipping From Multiple Points To Multiple Points With Excel Solver
- Knapsack Loading Problem in Excel Solver – Optimizing the Loading of a Limited Compartment
- Optimizing a Bond Portfolio With Excel Solver
- Travelling Salesman Problem in Excel Solver – Finding the Shortest Path To Reach All Customers
- Chi-Square Population Variance Test in Excel
- Analyzing Data With Pivot Tables
- SEO Functions in Excel
- Time Series Analysis in Excel
- VLOOKUP






No comments:
Post a Comment